Scaling the Edge Length

New version of fillygons code allowing us to specify the edge length. This is how we can build a triangle with a side length of $\sqrt{2}$ units.

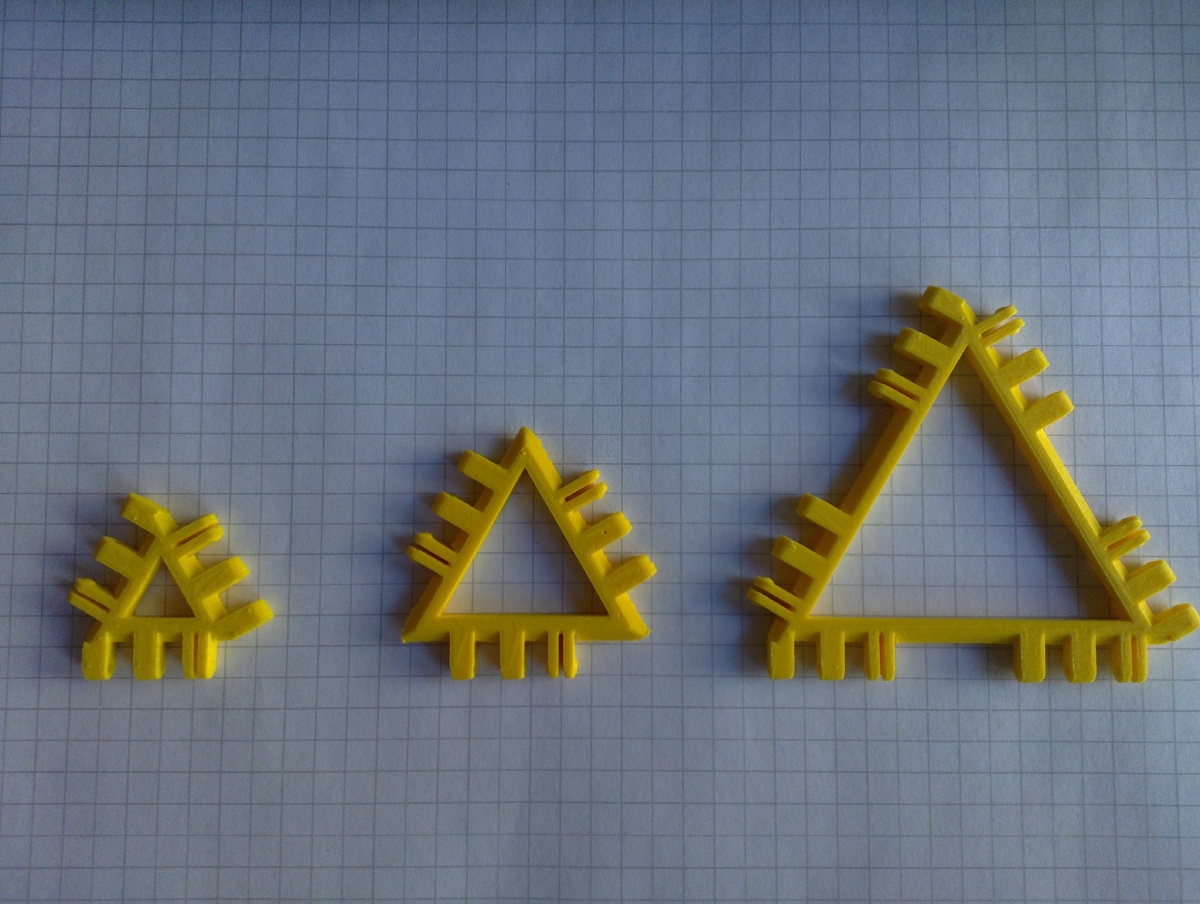
Example of these new fillygons include 3 gon sqrt2 (normal) and 4 gon sqrt2 (normal) which differ from models like 3 gon double (normal) and others. (The model 3 gon double (normal) is technically even a hexagon with three angles of $\frac{\pi}{3}$ and three angles of $\pi$.)

Such models are necessary for example for constructions like the one shown below. We start with a regular cube and cut away one corner. The emerging face (pink triangle) has sides of length $\sqrt{2}$.

Most Catalan solids even require fillygons having different edge lengths. The Pentagonal icositetrahedron for example can be built from the Pentagonal icositetrahedron (normal) model which posesses three shorter and two longer edges.